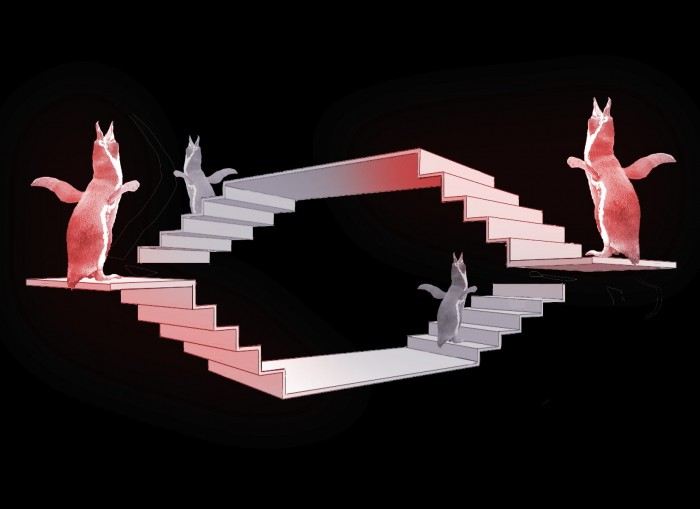
Here’s a new kind of never-ending stair (I think).  It’s like the famous never-ending staircase seen from above by M.C.Escher, called Ascending and Descending.  However, in this new staircase instead of figures doomed to go downstairs for ever we have penguins destined to walk away from us forever.  It’s based on the geometry of the object in my post on paradoxical size-constancy.

Here’s an animated version:

Like Escher’s famous impossible staircase, (and also as with the impossible tribar), the effect depends on our seeing a scene from a viewpoint from which points that would be at different distances from us seem to connect up. Here’s a view in more usual perspective of one configuration that would give rise to the ever receding staircase above. The trick depends not just on getting the alignment just right, but also on suppressing the usual perspective cues.  Size diminution with distance is the most important one.  The other is aerial perspective, in which contrast flattens out and colours get bluer with distance. I’ve put them both back below.

 

For more on staircases like Escher’s famous picture Ascending and Descending …..

Here’s a picture of penguins on a stripped down version of a never-ending staircase, like the one in M.C.Escher’s famous print Ascending and Descending. (Or just put the name Escher and that title into Google images to get stacks of versions). In my version, it’s penguins who will go on climbing the staircase forever.

Here’s how this kind of illusion works. In the middle picture below, the upper left bar seems to butt up to the upper right bar, and join it, even though the result is impossible for a 3-D configuration. On the left, it doesn’t quite butt up, and so appears, as in reality, both nearer to us and higher. (If you have the knack of viewing 3D picture pairs without a viewer, you’ll find the centre and left images can be fused to show the configuration in 3D).